Table of Contents (click to expand)
In free fall, it is the absence of a surface which causes you to become weightless.
In one of Einstein’s most renowned thought experiments, what he regarded as his “happiest thought”, he pictured a man falling in a free-falling elevator. The unfortunate man, he realized, if the elevator ceaselessly fell and didn’t meet the surface, would float, so he would feel weightless.
The implications of his thought, which may seem irrelevant right now, revolutionized our understanding of the universe. One implication though, which I’d like to address, was the possibility of fulfilling one of humankind’s deepest desires: time travel.

The question is, why should one feel weightless while falling in an uncorded elevator, as though floating in outer space, when one has not escaped the pull of Earth’s gravity? If the reader finds this surprising, it is likely so because he or she might believe that gravity is the force that imbues us with weight. This is an enormous – but common – misconception.
Recommended Video for you:
The Difference Between Mass And Weight
Of course, gravity doesn’t switch off when you leap from a window. If it weren’t for gravity, you wouldn’t accelerate. In fact, according to Newton’s second law of motion, acceleration is impossible to achieve without a force being involved: when you leap from a window (which, may I remind you, is not recommended), you don’t fall at a constant velocity, but instead accelerate, that is, you fall faster each second. Gravity is the force responsible for this acceleration. We denote the acceleration imparted by Earth’s gravity by ‘g’, and its value is 9.8 m/s².
As explained in a previous ScienceABC article:
“Mass” is defined as the amount of matter that an object contains, while the object’s “weight” represents the force that it exerts on another object purely under the influence of gravity. The magnitude of mass is unaffected by the forces of gravity; weight is the embodiment of this force itself.
In other words, gravity is responsible for the weight of a body.
According to Newton’s third law of motion, every action has an equal and opposite reaction. The weight of an object is actually the equal and opposite force, the normal force, which the Earth exerts on it, as a reaction to the force the object exerts on the Earth as it rests on its surface.
Weight is literally the measure of how strongly the Earth pulls the object because both forces are equal in magnitude. Weight, therefore, like any other force, is measured in Newton (N), the standard unit of force.
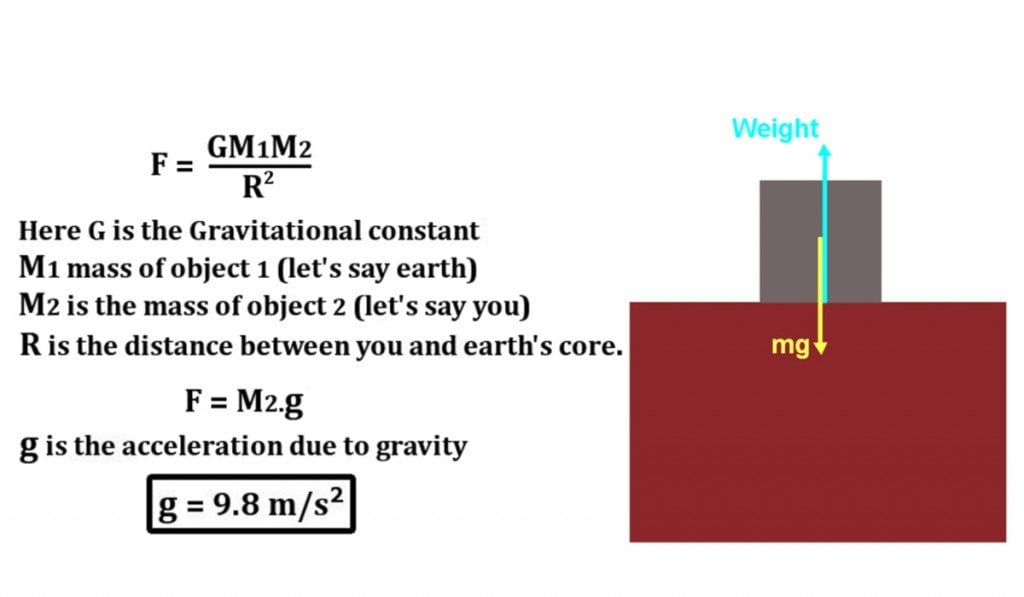
The normal force, or the reaction force, can be produced when there exists something upon which an object can rest/move. This would include the Earth’s surface or a lift’s floor.
If you were to leap from your window with a weighing scale attached to your feet, the machine’s needle would remain unperturbed throughout the fall because you are both falling at the same rate — just as you and the uncorded lift do — which prevents the generation of the normal force.
[adinserter block=”2″]

Lastly, while gravity affects mass — which is blindingly obvious as you, a lump of mass, fall or rather, as you are attracted towards an astronomically larger lump of mass, the Earth — it doesn’t change its magnitude. Your mass is 60 kg on Earth, the moon and every nook and cranny of the Universe. This is because mass is an inherent or purely a property of matter. The magnitude of the weight of an object, on the other hand, varies with the magnitude of the gravitational force with which it is pulled. Your 60 kg self, which weighs 882 Newtons on Earth, weighs merely 145.8 N on the moon, and approximately 2205 N on Jupiter.
This is because weight is merely the magnitude of the gravitational force with which a body pulls another body. Now, because the mass of a body is constant, its weight is solely a function of the rate at which it is accelerated — the acceleration due to gravity. If, like the moon, the body is less massive than Earth, then the force and therefore the weight generated would also be less in magnitude than it would be on Earth. The inverse would be true for a body that is more massive than Earth, such as Jupiter. The moon’s acceleration due to gravity is one-sixth, while Jupiter’s is more than twice the Earth’s, and therefore, so are the weights that are generated on their respective surfaces. Provided, of course, that you stand on them.













