What Is The Twin Paradox And How Does It Work?

The twin paradox is a thought experiment demonstrating the concept of time dilation in special relativity. In this experiment, one twin embarks on a rocket ship and travels near the speed of light while the other twin stays on Earth. Due to time dilation, the twin on the rocket ages less than the twin on Earth. 

Imagine having an identical twin (if you don’t have one already), meaning you are nearly the same age. 

Now, what if I told you that one of you can stay young while the other ages much quicker? 

You would probably say that it’s highly improbable, wouldn’t you? Well, let me be the first to tell you that it is possible and has even been scientifically verified.

Let’s dive into how this “twin paradox” plays out.

Recommended Video for you:

The Problem

Let’s imagine two twins, Humpty and Dumpty, who have just turned 20. Humpty discovers a rocket that can travel near the speed of light, so he decides to embark on space exploration, knowing that he can travel vast distances across the Universe with such a fast rocket. 

Humpty starts by traveling in a straight line at relativistic speed v to a distant location. He then slows down and turns back to return to his twin. Both Humpty and Dumpty have clocks with them. 

Dumpty observes that on the outward and return leg of the journey, Humpty’s clock advances slower. Humpty sees Dumpty’s clock move slower on his outward and return voyage. Each twin concludes that the other will age less than they will.

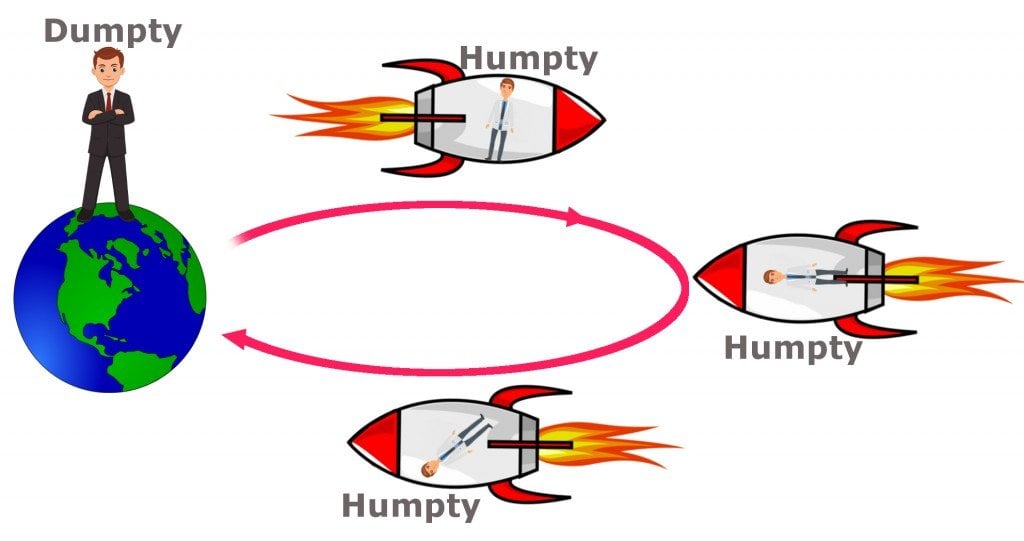
This is where the paradox arises, but we can scientifically prove that one twin will indeed age more significantly.

The twin paradox is a problem that involves relativistic time dilation, a concept from the special theory of relativity. Although the jargon may seem complex, the solution is quite intuitive and leads to a beautiful answer.

The Solution

To find the solution, we assume that both twins can keep track of each other’s clocks. The clock ticks only once per year, on the anniversary of the twins’ separation. They can also send an electromagnetic pulse to each other as an anniversary wish. The light from the telescope and the electromagnetic signal they send travel at the same speed.

Calculating from Dumpty’s frame of reference, assuming that the length of Humpty’s journey is 2.67 light-years for one leg of her journey, we can calculate the time of the journey to be 4 years (2.67 light-years/ 0.66c). 

So, from Dumpty’s perspective, Humpty’s trip time would actually be 8 years.

Calculating from Humpty’s frame of reference, we multiply the distance of her trip with the Lorentz Factor, which is 0.75 (calculated by plugging her velocity of 0.66c into the Lorentz Transformation equation and taking the inverse of the value). According to Dumpty, this 0.75 multiplied by the distance of 2.67 light years gives us approximately 2 light-years (to be more precise, it’s 2.0025 light years). Dividing the distance of 2 light-years with the speed at which she is traveling (0.66c), we get 3 years on each leg of the journey.

Therefore, from the time Humpty leaves Earth and returns, Dumpty would have aged 8 years, whereas Humpty would have aged only 6 years. This proves that when objects move closer to the speed of light, their experience is much slower than those who are not going at speeds relatively close to the speed of light.